History of Graph Theory:
The study of graphs, also known as graph theory, is an important part of many disciplines, including mathematics, engineering, physical, social, biological, and computer science, linguistics, and many others. The history of graph theory can be traced back to 1735, when Leonhard Euler, a Swiss mathematician, solved the Königsberg bridge problem.
A graph is a collection of points known as nodes or vertices that are linked together by a network of lines known as edges. Because graph theory is considered a branch of applied mathematics, it is no surprise that graph theory has been independently discovered numerous times.
Who invented graph theory:
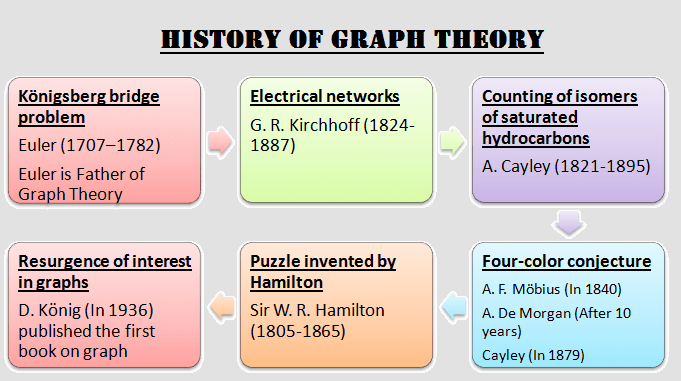
In 1736, Euler (1707–1782) published a paper in which he solved the Königsberg bridge problem, which gave birth to graph theory. Because graph theory is thought to have begun in 1736 with the publication of Euler’s solution to the Königsberg bridge problem, Euler became known as the “Father of Graph Theory.”
Nothing more was done in this field for the next 100 years.
- Then, in 1847, G. R. Kirchhoff (1824-1887) developed the tree theory in electrical networks for their applications. Kirchhoff’s research into electric networks led to the development of the fundamental concepts and theorems relating to trees in graphs.
- 10 years later, A. Cayley (1821-1895) thought about trees that arose from the counting of organic chemical isomers and discovered trees while trying to count the isomers of saturated hydrocarbons CnH2n+2.
- Two other milestones in graph theory were established around the time of Kirchhoff and Cayley.
- One milestone is the four-color conjecture, and
- the other milestone is a puzzle invented by Hamilton.
- Four-color conjecture:
According to the four-color conjecture, four colours are sufficient for colouring any atlas (a map on a plane) so that countries with common borders have different colours.
In 1840, the four-color problem is considered to have been first presented by A. F. Möbius (1790-1868) in his lectures.
About 10 years later, A. De Morgan (1806-1871) discussed this problem in London.
In 1879, Cayley published this problem in the “first volume of the Proceedings of the Royal Geographic Society”, and it quickly became well known. Following this, the famous Four-color conjecture gained fame and has remained popular ever since.
Even now, the four-color problem is by far the most famous unsolved problem in graph theory, inspiring a massive amount of research in the field.
- Puzzle invented by Hamilton:
In 1859, Sir W. R. Hamilton (1805-1865) invented a puzzle approach to graphs and sold it to a game manufacturer in Dublin for 25 guineas.
The puzzle was made up of a wooden regular dodecahedron (a polyhedron, with 12 faces all of which are regular pentagons, and 20 corners and 30 edges. 3 edges meet at each corner). The names of 20 important cities were marked on the corners. The goal of the puzzle was to find a path along the dodecahedron’s edges that passed through each of the 20 cities exactly once.
However the solution to this particular problem is simple, no one could yet find a necessary and sufficient condition for the existence of such a path (known as a Hamiltonian circuit) in any graph.
After this fruitful period, there was a half-century of relative inactivity.
- Then, in the 1920s, a resurgence of interest in graphs began. D. König was one of the pioneers during this time period. He organised the work of other mathematicians as well as his own and published the first book on the subject in 1936.
- The last 30 years have seen intense activity in graph theory — both pure and applied. A significant amount of research work has been done and continues to be done in this area. Thousands of papers and more than a dozen books have been published in the last decade.
- There have already been a great many rediscoveries of graph theory, in the twenty-first century.
(Source – Various books from the college library)
Tags: graph theory discrete mathematics, graph theory history
Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved
All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.




Be the first to comment