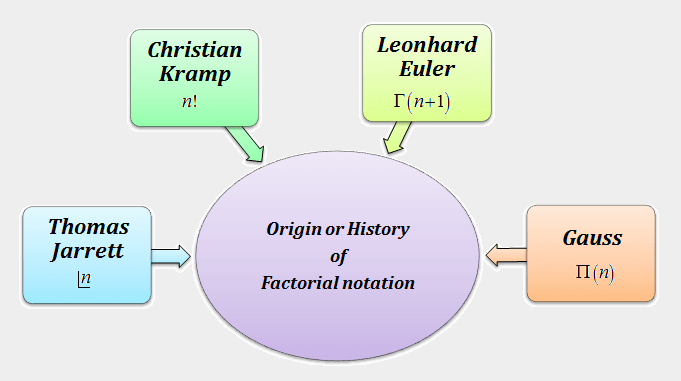
Origin or History of Factorial Notation

Origin or History of Factorial Notation:

We know that the n factorial is the number of permutations of n elements, or equivalently, the number of bijective functions from a set containing n elements to itself.

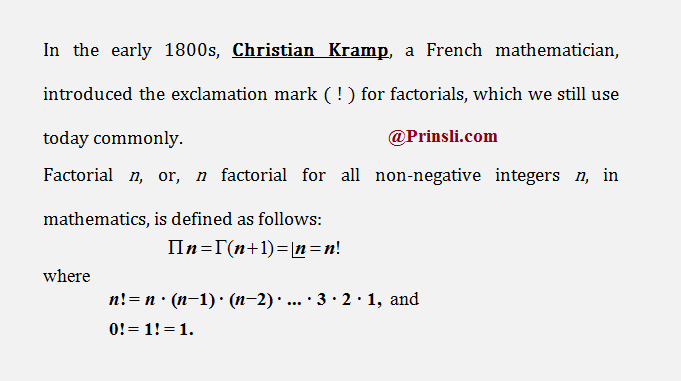
Factorial n is represented by several symbols, like n!,  , ∏(n) or Γ(n+1), where,

Γ(n+1) = ∏(n) =  = n! = n×(n−1)×(n−2)× . . . ×3×2×1 = n · (n−1) · (n−2) · … · 3 · 2 · 1.

For example,

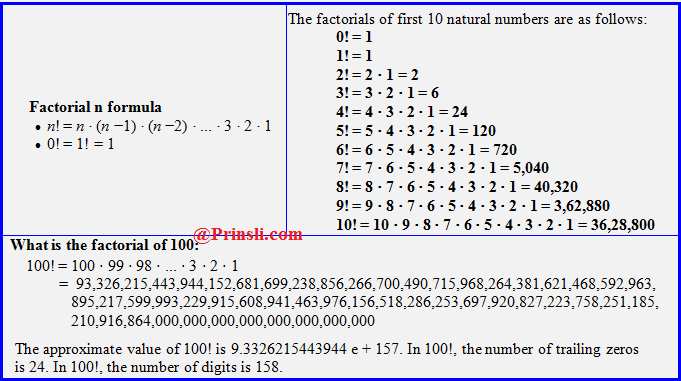
10! = 10×9×8×7×6×5×4×3×2×1= 3628800.

Γ(11) = 10×9×8×7×6×5×4×3×2×1 = 3628800.

∏(10) = 10×9×8×7×6×5×4×3×2×1 = 3628800.

Often our curiosity is to know that, where did the factorial sign come from? What is the history of the factorial symbol? Where did the factorial notation originate?

In this article, we will try to answer these questions.

The factorial is now globally denoted by an exclamation point, but this was not always the case. For example, Gauss used ∏(n) and Jarrett used “” for n!.

♦ Origin or History of Factorial notation “∟”:

First, in 1827, Thomas Jarrett introduced the notation “” for the n factorial.

In this notation, the factorial argument was half-enclosed by the left and bottom sides of a rectangle. So this notation is defined as follows:

= n · (n−1) · (n−2) · … · 3 · 2 · 1.

This notation was popular in Britain and America for some time, but it was not too satisfactory and fell out of use, perhaps because there were some typeset problems with this notation. The main problems with this notation are, to drawing a line under the character is uncomfortable and as well as, writing it by hand is also tough.

♦ Origin or History of Factorial notation “!”:

Fortunately, Christian Kamp created a better notation, the exclamation point n! for the n factorial, which we still use today.

In the early 1800s, Christian Kramp, a French mathematician, introduced the exclamation mark ( ! ) for factorial notation. In 1808, a little before Jarrett, he first mentioned this notation in his book “Élémens d’arithmétique Universelle”. In his book he wrote that he was using the very simple notation n! to represent the product of numbers decreasing from n to 1, that is,

n! = n · (n−1) · (n−2) · … · 3 · 2 · 1

Because he used combinatorial analysis in almost all of his demonstrations, this factorial notation is absolutely necessary for him.

But, there are still some minor problems in this notation.

For example, when factorials appear at the end of a sentence, sometimes they can appear unusual, such as 10!. In addition, if we include an exclamation point, such as, we like 100!! Then, in this case, there may be some confusion between the regular factorial and the double factorial, which is a completely different function.

Luckily, it is a good thing that the exclamation marks are not used by mathematicians in their writing, so this factorial notation is quite good.

♦ Origin or History of Factorial notation “Γ”:

In Many books, it is sometimes denoted by the symbol Γ(n+1), where

Γ(n+1) = n!

It is read as gamma n+1.

• In the 1720s, Daniel Bernoulli and Christian Goldbach realised that the definition of factorial was a very restrictive definition: in reality, it is defined only for non-negative integers, (that is, it is defined only for natural numbers and 0).

• In the late 1720s, Leonhard Euler was interested in extending the factorial to non-integer values.

In 1730, Leonhard Euler solved the problem of extending factorial to all real numbers.

In 1738, Euler introduced a generalisation of the factorial in the form of a function defined by a certain integral, which is now known as the Euler gamma function. So, the gamma function is a generalised factorial function in the sense that

Γ(n+1) = n!

for all non-negative integers n.

♦ Origin or History of Factorial notation “Π”:

In some books, factorial n is denoted by the product notation ∏(n) (where, ∏ is a greek letter corresponding to P and may be thought of as standing for the product), where

∏(n) = n!

This notation was introduced by Gauss.

Because of the difficulty in setting the notation in print, it is traditional to use the symbol ∏(n) for factorial n, more especially in Germany. This notation ‘∏(n)’ was given up after Legendre introduced the gamma-notation Γ(n+1), where ∏(n) = Γ(n+1).

In mathematics, generally, a factorial symbol is denoted by an exclamation point ( ! ), where factorial n is represented by that number n and an exclamation point !, that is, n!.

(Source – Various books from the college library)

Tags: Where did the notation ‘!’ originate? How is factorial symbol written? What does the factorial symbol (!) denote?

Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved

All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.