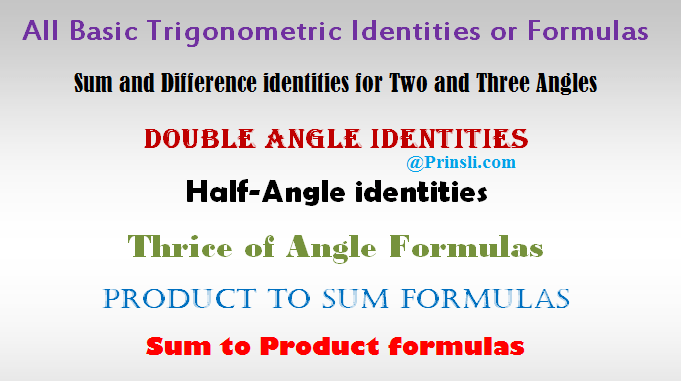
All Basic Trigonometric Identities Formulas

Lata Agarwal Trigonometry
all basic trigonometric identities or formulas, sum and difference identities for two and three angles, double angle identities, half-angle identities, thrice of angle formulas, product to sum formulas, sum to product formulas

All Basic Trigonometric Identities Formulas – In the previous article: Basic Trigonometry All Formulas; we have learned already all basic formulas of trigonometry, such as Pythagorean trigonometry identities, Reciprocal & Quotient Identities for trigonometric functions, Co-function identities (shifting angles), Even and Odd Angle Formulas, Trigonometry table formulas for angles, Trigonometrical ratios of Angle (90°+θ) in terms of those of θ for all values of θ, etc.

In this article, all basic Trigonometric Identities Formulas: such as Sum and Difference identities, Double Angle identities, Half-Angle identities, Thrice of Angle Formulas, Product to Sum formulas, Sum to Product formulas etc. are given. Students in Classes 10, 11, and 12 will benefit from learning and memorising these trigonometry math formulas in order to get success in this topic.

Read Also: Basic Concept of Trigonometry: Measurement of Angles

Trigonometric Identities:

Trigonometric identities are equations that contain trigonometric functions that hold true for every value of the variables involved.

♦ Pythagorean Identities in Trigonometry:

The Pythagorean Theorem is the source of some of the most commonly used trigonometric identities, such as:

1.   {\sin ^2}\theta + {\cos ^2}\theta = 1

2.   1 + {\tan ^2}\theta = {\sec ^2}\theta

3.   1 + {\cot ^2}\theta = {{\mathop{\rm cosec}\nolimits} ^2}\theta

Read Also: Difference between Trigonometric Identity and Trigonometric Equation

♦ The Bhaskaracharya Sum and Difference Identities in Trigonometry:

Sum and Difference Identities for Sine and Cosine functions in trigonometry for two angles A and B are as follows:

1.   \sin \left( {A + B} \right) = \sin A\cos B + \cos A\sin B

2.   \sin \left( {A - B} \right) = \sin A\cos B - \cos A\sin B

3.   \cos \left( {A + B} \right) = \cos A\cos B - \sin A\sin B

4.   \cos \left( {A - B} \right) = \cos A\cos B + \sin A\sin B

Sum and Difference Identities for Tan and Cot functions in trigonometry are as follows:

1.   \tan \left( {A + B} \right) = \frac{{\tan A + \tan B}}{{1 - \tan A\tan B}}

2.   \tan \left( {A - B} \right) = \frac{{\tan A - \tan B}}{{1 + \tan A\tan B}}

3.   \cot \left( {A + B} \right) = \frac{{\cot B\cot A - 1}}{{\cot B + \cot A}}

4.   \cot \left( {A - B} \right) = \frac{{\cot B\cot A + 1}}{{\cot B - \cot A}}

Sum Identities for three angles A, B and C for Sine, Cosine and Tan functions in trigonometry are as follows:

1.  \sin \left( {A + B + C} \right) 

= \sin A\cos B\cos C + \cos A\sin B\cos C + \cos A\cos B\sin C - \sin A\sin B\sin C

2.   \cos \left( {A + B + C} \right) 

= \cos A\cos B\cos C - \cos A\sin B\sin C - \sin A\cos B\sin C - \sin A\sin B\cos C

3.  \tan \left( {A + B + C} \right) 

= \frac{{\tan A + \tan B + \tan C - \tan A\tan B\tan C}}{{1 - \tan A\tan B - \tan B\tan C - \tan C\tan A}}

♦ Double Angle Identities in Trigonometry:

The double angle identities are just special cases of Bhaskaracharya’s Sum and Difference formulas, when A = B. A trigonometric expression can be written in terms of a single trigonometric function using the double angle identities. Here, trigonometrical ratios of angle 2A in terms of those of angle A for all values of A are given.

Double Angle Identities for Sine and Cosine functions in trigonometry are as follows:

1.   \sin 2A = 2\sin A\cos A

2.   (i)   \cos 2A = {\cos ^2}A - {\sin ^2}A

     (ii)   \cos 2A = 2{\cos ^2}A - 1

    (iii)   \cos 2A = 1 - 2{\sin ^2}A

Double Angle Identities for Sine and Cosine functions in terms of Tan function are as follows:

1.   \sin 2A = \frac{{2\tan A}}{{1 + {{\tan }^2}A}}

2.   \cos 2A = \frac{{1 - {{\tan }^2}A}}{{1 + {{\tan }^2}A}}

Double Angle Identities for Tan and Cot functions in trigonometry are as follows:

1.   \tan 2A = \frac{{2\tan A}}{{1 - {{\tan }^2}A}}

2.   \cot 2A = \frac{{{{\cot }^2}A - 1}}{{2\cot A}}

♦ Half-Angle Identities in Trigonometry:

The half-angle identities are also special cases of Bhaskaracharya’s Sum and Difference Formulas. Half-angle identities can be used to evaluate the trigonometrical function of an angle that is not on the unit circle. For example, we can find the value of the trigonometrical function of 15°, which is not on the unit circle, because 15° is half of 30°, which is on the unit circle. Here, trigonometrical ratios of angle A in terms of those of angle A/2 for all values of A are given.

Half-Angle Identities in terms of cos A are as follows:

1.  {\sin ^2}\frac{A}{2} = \frac{{1 - \cos A}}{2},   or    \sin \frac{A}{2} = \pm \sqrt {\frac{{1 - \cos A}}{2}}

2.   {\cos ^2}\frac{A}{2} = \frac{{1 + \cos A}}{2},   or    \cos \frac{A}{2} = \pm \sqrt {\frac{{1 + \cos A}}{2}}

3.   {\tan ^2}\frac{A}{2} = \frac{{1 - \cos A}}{{1 + \cos A}},   or    \tan \frac{A}{2} = \pm \sqrt {\frac{{1 - \cos A}}{{1 + \cos A}}}

Half-Angle Identities in terms of sin A are as follows:

1.  2\sin \frac{A}{2} = \pm \sqrt {1 + \sin A} \pm \sqrt {1 - \sin A}

2.  2\cos \frac{A}{2} = \pm \sqrt {1 + \sin A} \mp \sqrt {1 - \sin A}

♦ Thrice of Angle Identities in Trigonometry:

The thrice of angle identities, that is, trigonometrical ratios of angle 3A in terms of those of angle A for all values of A are as follows:

1.   \sin 3A = 3\sin A - 4{\sin ^3}A

2.   \cos 3A = 4{\cos ^3}A - 3\cos A

3.   \tan 3A = \frac{{3\tan A - {{\tan }^3}A}}{{1 - 3{{\tan }^2}A}}

♦ Sum-to-Product formulas or Identities in Trigonometry:

Sum to Product formulas or identities for two angles C and D in Trigonometry are as follows:

1.   \sin C + \sin D = 2\sin \left( {\frac{{C + D}}{2}} \right)\cos \left( {\frac{{C - D}}{2}} \right)

2.   \sin C - \sin D = 2\cos \left( {\frac{{C + D}}{2}} \right)\sin \left( {\frac{{C - D}}{2}} \right)

3.   \cos C + \cos D = 2\cos \left( {\frac{{C + D}}{2}} \right)\cos \left( {\frac{{C - D}}{2}} \right)

4.   \cos C - \cos D = 2\sin \left( {\frac{{C + D}}{2}} \right)\sin \left( {\frac{{D - C}}{2}} \right)

♦ Product-to-Sum formulas or Identities in Trigonometry:

Product to Sum formulas or identities for two angles A and Bin Trigonometry are as follows:

1.   2\sin A\cos B = \sin \left( {A + B} \right) + \sin \left( {A - B} \right)

2.   2\cos A\sin B = \sin \left( {A + B} \right) - \sin \left( {A - B} \right)

3.   2\cos A\cos B = \cos \left( {A + B} \right) + \cos \left( {A - B} \right)

4.   2\sin A\sin B = \cos \left( {A - B} \right) - \cos \left( {A + B} \right)

Tags: trigonometry formula, trigonometry formulas for class 10, trigonometry all formula, trigonometry formulas for class 11, inverse trigonometry formula, trigonometry table formula, trigonometry formulas for class 12, basic trigonometry formulas, trigonometric identities class 10, all trigonometric identities, trigonometric identities class 11, trigonometric identities formula, basic trigonometric identities, double angle identities trigonometry, half angle identities trigonometry, sum product formulas or identities, product sum formulas or identities

Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved

All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.

About Lata Agarwal 270 Articles
M.Phil in Mathematics, skilled in MS Office, MathType, Ti-83, Internet, etc., and Teaching with strong education professional. Passionate teacher and loves math. Worked as a Assistant Professor for BBA, BCA, BSC(CS & IT), BE, etc. Also, experienced SME (Mathematics) with a demonstrated history of working in the internet industry. Provide the well explained detailed solutions in step-by-step format for different branches of US mathematics textbooks.
Website Facebook LinkedIn

Related Articles

sexagesimal system centesimal circular systems angle trigonometry
Trigonometry

Sexagesimal, Centesimal, Circular Systems of Measuring Angles in Trigonometry

Lata Agarwal Trigonometry

Sexagesimal, Centesimal, Circular Systems of Measuring Angles in Trigonometry: Angles in geometry are measured in terms of a right angle… […]

difference between identity and equation, difference between trigonometric identity and trigonometric equation, difference between trig identity and trig equation, identity vs equation in math
Trigonometry

Difference between Trigonometric Identity and Trigonometric Equation

Lata Agarwal Trigonometry

Difference between Identity and Equation (Equation Vs Identity): Equation: An equation that holds true only for certain (some) values of the variable and false for others, is called a conditional equation. For example, an equation: […]

trigonometry measurement of angles
Trigonometry

Basic Concept of Trigonometry: Measurement of Angles

Lata Agarwal Trigonometry

Basic Concept of Trigonometry: Measurement of Angles – Definitions of Ray, Angle, Degree, Radians, Gradians, … […]

Be the first to comment

Leave a Reply

Your email address will not be published.


*