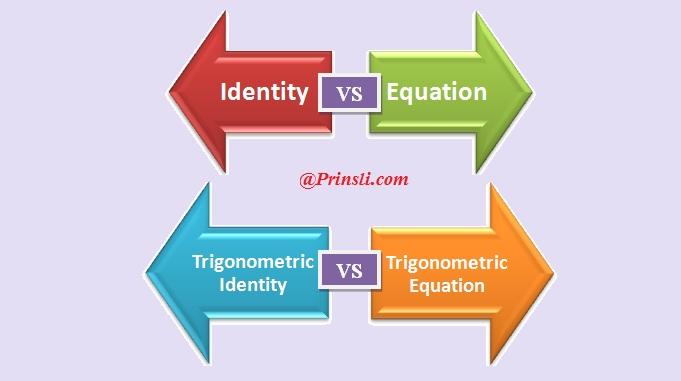
Difference between Identity and Equation (Equation Vs Identity):

Equation:

An equation that holds true only for certain (some) values of the variable and false for others, is called a conditional equation. For example, an equation:

x² + 9x =10;

is true only if x = 1 or x = −10, so this equation is a conditional equation.

Identity:

Some equations are true for all legitimate (valid) values of the variables, such types of equations are called identities. As a result, an identity is an equation that holds true for all valid (legitimate) values of the variables. For example, an equation:

x² + 9x = x (x + 9);

is true for all values of x, so it is an identity.

In other words, an equation that is true for all values of the variable (s) involved, is called an identity.

In identity, the expressions on both sides of the equal sign are equivalent expressions, because they have the same value for all values of the variable.

Examples of equations and identities:

1. Equation, 2x + 3x = 5x is obviously true for all values of the variable x, so this equation is an identity.

2. Equation x² + 3x =10 is true only if x = 2 or x = −5, so this equation is a conditional equation.

3. Similarly, x²−4=(x−2) (x+2) is an algebraic identity as it is satisfied by every value of the variable x.

4. Equations: 3(x + y) =3x + 3y and (x + 1)² = x² + 2x + 1; are also indentities because these are true for all values of the variable x.

Important Note:

1. Remember that depending on the values of any variables involved, an equation can be true or false. For example, the equation x² −9 =0 is true only if x = −3 or x = 3.

2. When we solve a conditional equation, we are finding the variables’ values that make the equation true.

Read Also: Basic Trigonometry All Formulas

Difference between Trigonometric Identity (trig identity) and Trigonometric Equation (trig equation):

Trigonometric Identity (trig identity):

An equation involving trigonometric ratios of an angle θ (say) is said to be a Trigonometric Identity (trig identity) if it is satisfied for all values of θ for which the given trigonometric ratios are defined.

A trigonometric identity is an equation which is true for all inputs (such as angles, θ.) For example,

sin² θ+ cos² θ=1

is a trigonometric identity, because it is true regardless of the choice of θ.

Read Also: Trigonometric Identities Formulas

Trigonometric Equation (trig equation):

Unlike an identity, a Trigonometric Equation (trig equation) may not necessarily be true for all angles θ. For example,

sin θ = cos θ

is a trigonometric equation, because it is true only for certain (some) values of θ, not all.

In general, we want to solve for the variable θ in a trigonometric equation. However, it is important to find all solutions when “solving” an equation.

Trigonometric identities describe the equality of related trigonometric expressions, whereas trigonometric equations require us to find the specific values of the variables that make two expressions equal.

Note that:

1. To prove that an equation is not an identity, we need to find only one value of the variable for which the equation is false.

2. But, to prove that a given equation is an identity, it is not sufficient to show that the equation is true for one or even a dozen different values of the variable, because the equation must hold true for all valid values of the variable. In this case, we must use algebraic methods to prove that an equation is an identity because we cannot check all values of the variable numerically.

Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved

All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.