Introduction of factorial & factorial notation, History of factorial

Introduction of factorial notation:

The factorial function is one of the most famous functions in several areas of mathematics, and it is used notably in combinatorics, algebra, and mathematical analysis. The factorial of a natural number n is represented by the symbol n!. The factorial notation is a symbol for representing a multiplication operation. But, it is much more than a symbol.

Since the 1200s, factorials have been used to count permutations. When arranging objects, factorial notation plays a vital role.

We know that the number of permutations of n elements is ⁿP_n=n!. Also, we know that the number of bijections from a set containing n elements to itself is also n!. Thus, we can say that the n factorial, is the number of permutations of n elements, or equivalently, the number of bijective functions from a set containing n elements to itself.

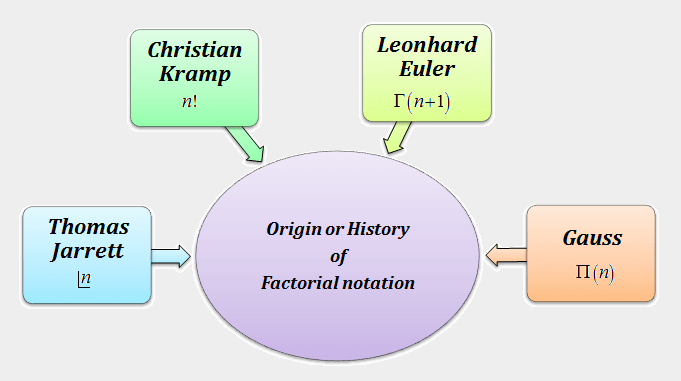
In 1800, the term “factorial” (originally French: factorielle) was firstly introduced by Louis François Antoine Arbogast referring to a broader concept of products of arithmetic progressions in his work. The “factor” that this name refers to are the words of the multiplication formula for the factorial.

So, in general, Louis François Antoine Arbogast (1759 – 1803), a French mathematician, is credited as the first to introduce the concept of the factorial.

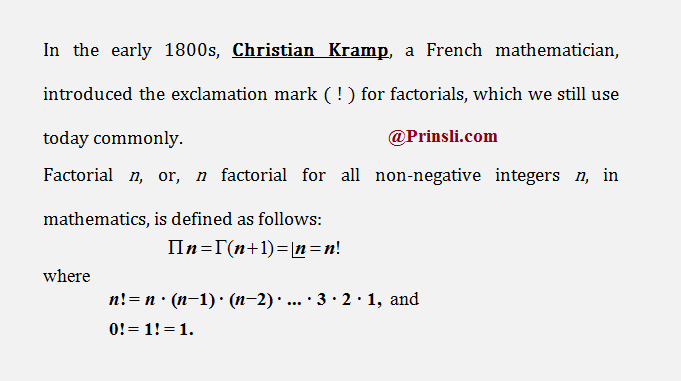
In the early 1800s, Christian Kramp, a French mathematician, introduced the exclamation mark ( ! ) for factorials.

Christian Kramp familiarized the notation, the exclamation mark n!, for factorial n, which we still use today commonly.

Factorial n, or, n factorial for all non-negative integers n, in mathematics, is defined as follows:

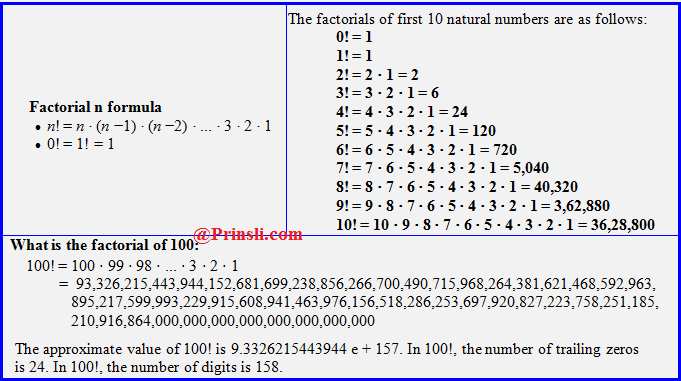
n! = n · (n−1) · (n−2) · … · 3 · 2 · 1, and 0! = 1.

Origin or History of factorial:

The concept of factorials has been independently arisen and developed in several ancient cultures, especially in the canonical works of Jain literature in Indian mathematics and in the Talmudic book Sefer Yetzirah in Jewish mystics.

Most of the mathematics of the factorial function was developed in the late 18th and early 19th centuries.

♦ Factorial in Indian Mathematics:

The contributions of Indian mathematicians in the evolution of factorial concepts are as follows:

In Indian mathematics, one of the earliest known descriptions of factorials appears in the Anuyogadvāra-sūtra, one of the canonical works of Jain literature, with dates ranging from 300 BCE to 400 CE.

In 6th-century CE, Jinabhadra, a Svetambara Jain monk, also described the product rule for permutations.

Since at least 1150, Hindu scholars have been using factorial formulas, when the Indian mathematician Bhaskara II wrote Lilavati, a treatise on mathematics.

He mentioned factorials in his book Lilavati, in connection with the problem of how many ways ShriHari Bhagwan Vishnu could hold his 4 attribute objects (Shankh – conch shell, Chakra – discus, Gada – mace, and Saroja – lotus flower) in his four hands.

Likewise, a similar problem of how many ways Bhagwan Shiv Shambhu could hold his 10 attribute objects (Pasha – rope, Ankusha – elephant’s hook, Ahi – serpent, Damru – drum, Kapal – skull, Trishul – trident, Khatvang – club, Shakthi – dagger, Shar – arrow, and Chap – bow) in his 10 hands.

Indian mathematician Bhaskara II wrote Lilavati — A mathematical problem based on Bhaskaracharya’s Lilavati. How many different forms of Shiva and Vishnu can be formed from the given combination of objects held in each of their hands?

♦ Factorial in Middle East Mathematics:

The contributions of Middle-east mathematicians in the evolution of factorial concepts are as follows:

From the Talmudic period (200 to 500 CE), the Jewish mystics book of creation Sefer Yetzirah lists factorials up to factorial 7 as part of an investigation into the number of words that can be formed using the Hebrew alphabet.

In the 8th-century, factorials were also studied by Arab grammarian Al-Khalil ibn Ahmad al-Farahidi for similar reasons. Ibn al-Haytham was the first Arab mathematician to formulate Wilson’s theorem connecting factorials with the prime numbers.

♦ Factorial in European Mathematics:

The significant contributions of European mathematicians in the evolution of factorial concepts are as follows:

There is no direct evidence of the study of factorials in ancient Greece, despite the fact that Greek mathematics included some combinatorics, and Plato was well-known for using the number 5040 (a factorial) as the population of an ideal community in part, due to its divisibility properties.

Rather than, Jewish scholars, such as Shabbethai Donnolo, in Europe, was the first to work on factorials, interpreting the Sefer Yetzirah passage.

From the late 15th century onward, factorials became a topic of study for western mathematicians.

In a treatise published in 1494, Luca Pacioli, an Italian mathematician, calculated factorials up to 11! in response to a problem involving dining table arrangements.

In 1603, commentary on Johannes de Sacrobosco’s work, Christopher Clavius discussed factorials.

In the 1640s, Marin Mersenne, a French polymath, published large (but not totally accurate) factorial tables, up to 64!, based on Clavius’ work.

In 1676, in a letter to Gottfried Wilhelm Leibniz, first Isaac Newton formulated the power series for the exponential function with the reciprocals of factorials for its coefficients.

In 1677, Fabian Stedman, a British author, described the use of factorials in change ringing, a musical art that involves the ringing of many tuned bells.

In 1685, John Wallis’ treatise gives extensive coverage on factorials.

In 1721, Abraham de Moivre conducted a study of their approximate values for large values of n.

In 1729, James Stirling stated Stirling’s approximation (an approximation for factorials) in a letter to De Moivre. At the same time, Daniel Bernoulli and Swiss mathematician Leonhard Euler (1707-1783) formulated the continuously enhanced version of the factorial function to the gamma function.
In an 1808 text on number theory, Adrien-Marie Legendre (1752-1833) included Legendre’s formula, which describes the exponents in the factorization of factorials into prime powers.

(Source – Various books from the college library)

All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.

About Lata Agarwal 270 Articles

M.Phil in Mathematics, skilled in MS Office, MathType, Ti-83, Internet, etc., and Teaching with strong education professional. Passionate teacher and loves math. Worked as a Assistant Professor for BBA, BCA, BSC(CS & IT), BE, etc. Also, experienced SME (Mathematics) with a demonstrated history of working in the internet industry. Provide the well explained detailed solutions in step-by-step format for different branches of US mathematics textbooks.