
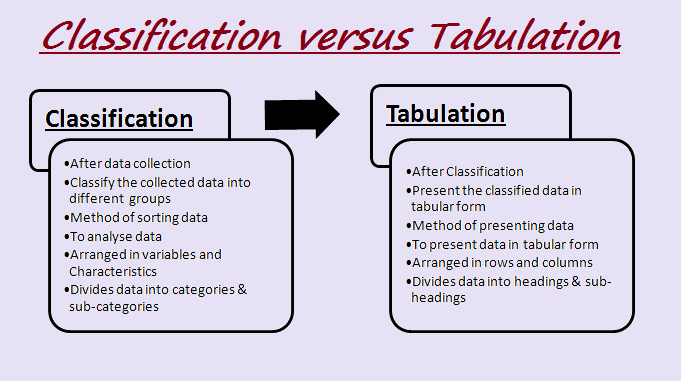
Meaning and Definition of Average In Hindi
केंद्रीय प्रवृत्ति की माप- मापनीय विशेषताएं जैसे वर्षा, ऊंचाई, जनसँख्या का घनत्व, आय का स्तर या आयु वर्ग में विभिन्नताएं पायी जाती हैं. यदि हमें इन्हें समझना है तो हमें क्या करना होगा? उसके लिए हमें एक ऐसे मूल्य या मान की जरूरत होगी, जो पर्यवेक्षणों के समूह का सबसे अच्छा प्रतिनिधित्व करता हो (We need a value that best represent the set of observations).
यह एकल मान आमतौर पर वितरण के किसी भी छोर पर स्थित होने की बजाय उसके केंद्र में स्थित होता है. वितरण का केंद्र ज्ञात करने वाली सांख्यिकीय विधियों को केंद्रीय प्रवृत्ति की माप (Measure of Central Tendency) कहा जाता है. यह संख्या सारे आंकड़ों के समूह की प्रतिनिधि संख्या होती है.
जैसे- किसी कक्षा में छात्रों द्वारा किसी परीक्षा में प्राप्त किये गए औसत अंक, किसी क्षेत्र विशेष की औसत वर्षा, किसी कारखाने में औसत उत्पादन, किसी देश के लोगों की औसत आय आदि.
केंद्रीय प्रवृत्ति की मापों को सांख्यिकीय औसत (Statistical Averages) के नाम से भी जाना जाता है. केंद्रीय प्रवृत्ति के कई माप हैं, जिनमें माध्य, माध्यिका और बहुलक (Mean/Average, Median, and Mode) सबसे महत्वपूर्ण हैं.
माध्य (Mean/Average)
माध्य या औसत वह मान है जो सभी मानों या मूल्यों के योग को प्रेक्षणों की कुल संख्या से भाग देकर प्राप्त होता है.
जैसे मान लीजिये कि 6 परिवारों की मासिक आय (रुपये में) निम्नलिखित है-
1400, 1500, 1600, 1525, 1625, 1630.
यहां पर इन परिवारों की औसत आय प्राप्त करने के लिए (या इन सभी संख्याओं का माध्य ज्ञात करने के लिए) सभी परिवारों की आय को एक साथ जोड़कर, उसे (योग को) परिवारों की संख्या से विभाजित कर दिया जाता है-
1400+1500+1600+1525+1625+1630
————————————————
6
= 1547 रुपये
माध्य का अर्थ और परिभाषा (Meaning and Definition of Mean/Average)
माध्य किसी समंक श्रेणी (Data Series) का एक ऐसा विशिष्ट मूल्य है, जिसके आसपास अन्य समंकों के केंद्रित होने की प्रवृत्ति पाई जाती है. यह श्रेणी के सीमांत पदों के बीच स्थित एक ऐसा अंक है जो वितरण के मध्य भाग में मूल्यों के जमाव की जानकारी देता है. इस दृष्टि से इसे केंद्रीय प्रवृत्ति का माप भी कहा जाता है, क्योंकि व्यक्तिगत चर मूल्यों का जमाव अधिकतर उसके आसपास ही होता है.
अतः माध्य संपूर्ण वितरण की अभिव्यक्ति का एकमात्र सार्थक प्रतिनिधि मूल्य है. यह एक ऐसी सरल और संक्षिप्त संख्या है जो समंक श्रेणी की मुख्य विशेषताओं पर प्रकाश डालती है.
(An average is a significant single expression representative of the whole distribution. Average is such a simple and concise number that throws light on the main features of the data series.)
• नीचे हम माध्य की प्रमुख परिभाषाओं का अध्ययन करेंगे-
क्लार्क और शकाडे के अनुसार-
“माध्य, समंकों के सम्पूर्ण समूह का विवरण प्रस्तुत करने के लिए, कोई अकेला अंक प्राप्त करने का प्रयास है.”
(Average is an attempt to find one single figure to describe whole group of figures.)
प्रो. क्रोक्सटन और काउडेन के अनुसार-
“माध्य, समंकों के विस्तार के अंतर्गत स्थित एक ऐसा मूल्य है, जिसका प्रयोग श्रेणी के सभी मूल्यों का प्रतिनिधित्व करने के लिए किया जाता है. चूंकि माध्य समंकों के विस्तार के अंतर्गत ही होता है, इसलिए इसे केंद्रीय मूल्य का माप भी कहा जाता है.”
(An average is a single value within the range of the data that is used to represent all the values in the series. Since an average is some where within the range of the data, it is sometimes called a major of central value.)
सिम्पसन और काफ्का के अनुसार-
केंद्रीय प्रवृत्ति की माप एक ऐसा प्रतिरूपी मूल्य है जिसके चारों ओर अन्य संख्याएँ संकेन्द्रित होती हैं.”
(A measure of central tendency is a typical value around which other figure congregate.)
उपरोक्त परिभाषाओं से यह स्पष्ट है कि कोई ऐसी अकेली संख्या जो श्रेणी के सभी समंकों का प्रतिनिधित्व करती हो, माध्य कहलाती है. माध्य में वे सभी विशेषताएं होती हैं, जो श्रेणों के अन्य मूल्यों में पायी जाती हैं. इसे अलग-अलग नामों से पुकारा जाता है, जैसे केंद्रीय प्रवृत्ति की माप, सांख्यिकीय माप, प्रतिनिधि मूल्य इत्यादि. इसे उसी इकाई में व्यक्त किया जाता है जिसमें प्रारम्भिक समंक संग्रहित होते हैं.
Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved
All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.



Be the first to comment