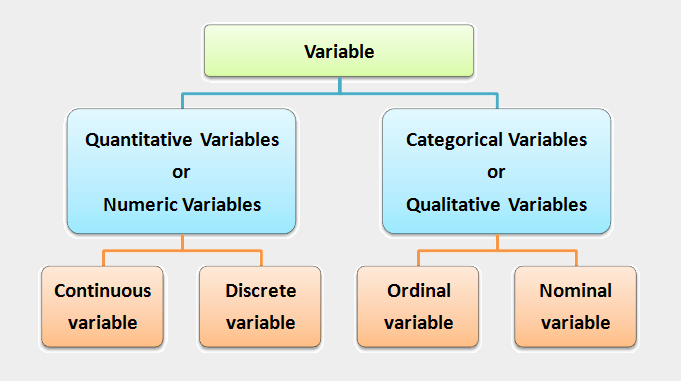
Quantitative Variables or Numerical Variables:

Numerical variables, such as counts, percents, numbers, etc. are quantitative variables. If observations on a variable take numerical values that represent distinct magnitudes of the variable, it is called a quantitative variable. In other words, a quantitative variable is a variable that differs in quantity. For a quantitative variable, each value it can take is a number, and so quantitative variables are classified as either discrete or continuous variables.

Examples of Quantitative Variables or Numerical Variables:

Daily high temperature, the number of rainy days, age, annual income, the number of years of education completed, the number of family members, etc. are all examples of quantitative variables. The following examples are also quantitative variables.

• GPA (Grade Point Average) in high school (e.g. 5.0, 4.2, 1.1).
• The number of pets you own (e.g. 1, 3, 5).
• The number of cousins you have (e.g. 0, 8, 18).
• The number of stars in a galaxy (for example, 200, 1301, or one trillion).
• The number of lottery tickets sold on average (e.g. 45, 989, 4 million).
• The balance of your bank account (for example, 967, and 500, 4222).

General rule for Quantitative Variables or Numerical Variables: 

You are working with a quantitative variable, if you can add these variables, or if you can get a meaningful result by subtracting the values of two variables.

• For example, a GPA (Grade Point Average) of 2.4 and a GPA of 5.0 can be added together (2.4 + 5.0 = 7.4), implying that it is quantitative.
• On the other hand, grades/ranks of A, B, or C, cannot be added together until they are converted to numbers, hence A, B, and C are not quantitative.

Important Remarks on Quantitative Variables:

Numerical values must represent different magnitudes in the definition of a quantitative variable because quantitative variables measure “how much” of something (that is, quantity or magnitude). By using quantitative variables, arithmetic summaries, such as averages, can be found.

However, because some numerical variables, for example, bank account numbers, area codes, etc. do not differ in quantity, so they are not quantitative variables. A bank, for example, would be interested in the average loan sizes issued to its customers, but for its loan accounts, an “average” bank account number is nonsensical.

The major characteristics of a quantitative variable are described via graphs and numerical summaries. The center and variability (sometimes known as spread) of the data are important characteristics to describe for quantitative variables. For example, what is a typical annual quantity of snowfall? Is there a lot of variation year to year?

Types of Quantitative variables:

For a quantitative variable, each value it can take is a number, and so quantitative variables are classified as either discrete or continuous variables. That is, there are two types of Quantitative variables:

(i) Discrete variables,

(ii) Continuous variables.

(i) Discrete Variables:

A quantitative variable is discrete if its possible values make a collection of separate numbers, such as 0, 1, 2, 3,… Typically, a discrete variable is a count (“the number of…”). So, a fraction between one value and the next closest value cannot be taken by a discrete variable.

A discrete variable is a quantitative variable that has no values in between the two given values. Any variable that begins with the statement “the number of…” is discrete. The possible values are the counting numbers such as 0, 1, 2, 3, 4,…, that is, the discrete variable’s output is a count.

In other words, a discrete variable is a quantitative variable with a certain number of possible values. Here, observations can be taken a value based on a count of distinct whole numbers. 

Examples of Discrete Variables:

The number of pets in a household (e.g. 1, 3, 5), the number of cousins you have (e.g. 0, 8, 18), etc are all discrete variables because these are measured in whole units. The following examples are also discrete variables.

• The number of children in a family.
• The number of foreign languages in which a person is fluent.
• The number of business sites.
• The number of registered cars.

(ii) Continuous Variables:

A continuous variable is a quantitative variable which can have any value within certain limits. It can take all possible values (integral, as well as fractions or decimals) within a specified range.

If a variable may take on an infinite number of real values within a given interval, it is said to be continuous. A continuous variable has a continuum of infinitely many possible values (such as height, weight, time, distance, etc.).

For instance, consider the height of a student. The height between 4 and 6 feet, the number of possible values is theoretically infinite. A student may be 5.6321748755 … feet tall, so height is a continuous variable. Whereas the number of cars in a street is not a continuous variable.

In other words, the collection of all the possible values of a continuous variable does not consist of a set of separate numbers but, rather, an infinite region of values. That is, continuous variables can take any value in between the two given values.

In other words, the continuous variables are those that are in units of measurement, which can be divided into infinite gradation such as temperature (decimal of a degree), length (decimal of an inch), etc. That is, the continuous variable is a variable with infinite number of values, like “time” or “weight”.

A variable is continuous if for every pair of values of the variable, a value exactly mid-way between them is meaningful. For example, two values for the time taken for a travel can be 2 and 3 hours, the mid-way value would be 2.5 hours which makes sense. However, for a number of pets, suppose you have a pair of values 5 and 8, the midway value would be 6.5 pets, which does not make sense.

Examples of Continuous Variables:

For example, the amount of time it takes to complete a task is a continuous variable, because the amount of time needed to complete a task could take the value 2.495731… hours. Continuous variables can take on an unlimited number of various values. Height, time, age, temperature, weight, etc. are all also continuous variables because these are measured in an infinite region of values.

(Source – Various books from the college library)

Tags: Which variables are discrete continuous? Is qualitative variable discrete or continuous? Are all discrete and continuous variables quantitative in nature? Are discrete variables quantitative or qualitative? quantitative discrete or continuous

Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved

All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.