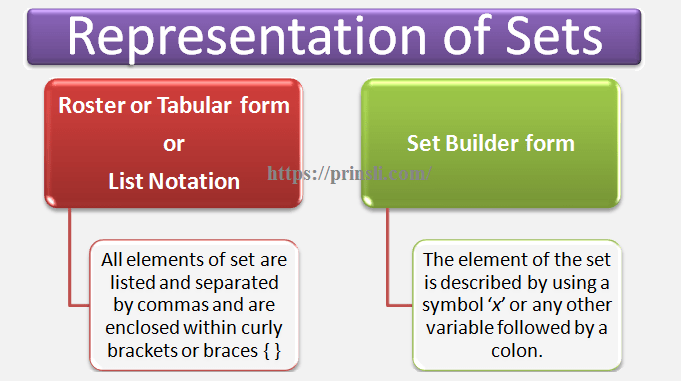
Representation of Sets in Mathematics: In the set representation, curly brace notation { } is used to enclose the elements. To represent a set, various methods can be used. The following are two common methods for representing a set:
1. Roaster form or tabular form method.
2. Set Builder method.
1. Roster or Tabular form or List notation –
In roster form, all elements of the set are listed, the elements are separated by commas, and all these elements are enclosed in curly brackets or braces { }.
For example, consider the set of digits of numbers 0, 1, 2, 3, 4, 5, 6, 7, 8, and 9. If this set is denoted by the symbol D, then we can write this set in Roster form, as follows:
D = {0, 1, 2, 3, 4, 5, 6, 7, 8, 9}.
Examples:
- The set of all even positive integers less than 8 is described in Roster form as {2, 4, 6}.
- The set of all leap years between 1995 and 2015 is described in Roster form as {1996, 2000, 2004, 2008, 2012}.
In these examples, the elements within the braces are written in increasing order. This could be in any order, including descending or random, because, for a set represented in the Roster Form, the order does not matter.
In addition, when representing the sets, multiplicity is ignored. For example, if A represents a set of all the letters in the word ‘mathematics’, the proper Roster form representation would be,
A = {m, a, t, h, e, i, c, s} = {a, c, e, h, i, m, s, t},
But,
A ≠ {m, a, t, h, e, m, a, t, i, c, s}, or A ≠ {a, a, c, e, h, i, m, m, s, t, t}
2. Set-Builder form –
In the Set-Builder form, all of the set’s elements must share a single property in order to be members of that set; that is, all of the elements of the set share a common attribute. This property does not apply to elements that are not members of the set.
In this method of set representation, the element of the set is specified using the symbol ‘x’ or any other variable followed by a colon. The sign ‘:’ or ‘|’ is used to indicate such that, after which we write the property owned by the set’s element and enclose the entire description in braces. The colon denotes ‘such that,’ while the brackets denote ‘set of all.‘
That is, in the Set-Builder form,
S={ x : property possessed by the set’s element},
where,
- ‘x’ is a symbolic representation that is used to describe the element.
- ‘:’ means ‘such that’
- ‘{ }’ means ‘the set of all’
For example, consider the set of digits of numbers 0, 1, 2, 3, 4, 5, 6, 7, 8, and 9. If this set is denoted by the symbol D, then we can write this set in Set-Builder form, as follows:
D = {x : x is a digit}, or D={x | x is a digit}.
So, it is read as ‘the set of all x such that x is a digit’.
Examples:
- The set of all even positive integers less than 10 is described in Set-Builder form as
S = {x : x < 10, x is an positive integer}.
It is read as ‘the set of all x such that x is an even positive integer and less than 10’.
- The set of all even prime numbers is described in Set-Builder form as
S={ x: x is an even prime number}.
It is read as ‘the set of all x such that x is an even prime number’.
समुच्चय का निरूपण (Representation of Sets in mathematics in Hindi):
समुच्चय को निरूपित करने के लिए, समुच्चय के सभी अवयवों को मझले कोष्ठक { } अंदर लिखते है। किसी समुच्चय को निरूपित करने के लिए, विभिन्न तरीकों का इस्तेमाल किया जा सकता है। समुच्चय को निरूपित करने की दो सामान्य विधियाँ निम्नलिखित हैं:
1. रोस्टर या सारणीबद्ध रूप विधि।
2. समुच्चय निर्माण रूप।
1. रोस्टर या सारणीबद्ध रूप विधि –
रोस्टर रूप में, समुच्चय के सभी तत्वों (अवयवों) को, अर्ध-विराम द्वारा अलग करते हुए, सूचीबद्ध किया जाता है, और इन सभी अवयवों को मझले कोष्ठक { } के अंदर लिखते हैं।
उदाहरण के लिए, संख्या 0, 1, 2, 3, 4, 5, 6, 7, 8 और 9 के अंकों के समुच्चय पर विचार करें। यदि इस समुच्चय को प्रतीक D से दर्शाया जाए, तो हम इस समुच्चय को रोस्टर रूप में इस प्रकार लिख सकते हैं:
D = {0, 1, 2, 3, 4, 5, 6, 7, 8, 9}।
उदाहरण:
- 8 से कम सभी सम धनात्मक पूर्णांकों के समुच्चय का रोस्टर रूप में वर्णन {2, 4, 6} द्वारा किया जाता है।
- 1995 और 2015 के बीच के सभी लीप वर्षों के समुच्चय का रोस्टर रूप में वर्णन {1996, 2000, 2004, 2008, 2012} द्वारा किया जाता है।
इन उदाहरणों में, मझले कोष्ठक के भीतर के तत्वों को बढ़ते क्रम में लिखा गया है, लेकिन यह अवरोही या अनियमित सहित किसी भी क्रम में हो सकता है, क्योंकि रोस्टर रूप में लिखे गए समुच्चय के लिए अवयवों के क्रम का कोई महत्व नहीं होता है।
इसके अलावा, समुच्चय को निरूपित करते समय, अवयवों की बहुलता को नजरअंदाज कर दिया जाता है, अर्थात किसी भी अवयव को दोबारा नहीं लिखते है, अर्थात प्रत्येक अवयव दूसरे से भिन्न होता है। उदाहरण के लिए, ‘mathematics’ शब्द के सभी अक्षरों के लिए रोस्टर रूप में समुच्चय निम्न प्रकार से लिखेंगे-
A = {m, a, t, h, e, i, c, s} = {a, c, e, h, i, m, s, t},
परंतु,
A ≠ {m, a, t, h, e, m, a, t, i, c, s}, या, A ≠ {a, a, c, e, h, i, m, m, s, t, t}
2. समुच्चय निर्माण रूप –
समुच्चय निर्माण रूप में, समुच्चय के सभी अवयवों में एक सर्वनिष्ठ (समान) गुणधर्म होता है। यह गुण उन अवयवों में नहीं होता जो समुच्चय के अवयव नहीं हैं।
समुच्चय निरूपण की इस पद्धति में, समुच्चय के अवयवों का वर्णन करने के लिए प्रतीक ‘x’ या किसी अन्य चर का उपयोग करते है और उसके बाद कोलन के चिन्ह ‘:’ या ‘|’ को लिखते है, कोलन के चिन्ह के बाद समुच्चय के अवयवों के विशिष्ट गुणधर्म लिखते हैं और इस पूरे विवरण को मझले कोष्ठक { } के अंदर लिखते हैं। कोलन ‘जहाँ x’ को दर्शाता है, जबकि कोष्ठक ‘सभी x का समुच्चय’ को दर्शाता है।
यानी समुच्चय निर्माण रूप में,
S={ x : समुच्चय के अवयवों का विशिष्ट गुणधर्म},
जहां पर,
- ‘x’ एक प्रतीक है जिसका उपयोग समुच्चय के अवयवों का वर्णन का वर्णन करने के लिए किया जाता है।
- ‘:’ का अर्थ है ‘जहाँ x’
- ‘{ }’ का अर्थ है ‘सभी x का समुच्चय’
उदाहरण के लिए, संख्या 0, 1, 2, 3, 4, 5, 6, 7, 8 और 9 के अंकों के समुच्चय पर विचार करें। यदि इस समुच्चय को प्रतीक D से दर्शाया जाए, तो हम इस समुच्चय को समुच्चय निर्माण रूप में इस प्रकार लिख सकते हैं:
D = {x : x एक अंक है}, या
D = {x | x एक अंक है}
इस समुच्चय को निम्न प्रकार से पढ़ते है-
‘सभी x का समुच्चय जहाँ x एक अंक है’।
उदाहरण:
- 10 से कम सभी सम धनात्मक पूर्णांकों के समुच्चय को समुच्चय निर्माण रूप में निम्न प्रकार से लिखेंगे:
S = {x : x <10, x एक सम धनात्मक पूर्णांक है}।
इस समुच्चय को निम्न प्रकार से पढ़ते है- ‘सभी x का समुच्चय, जहाँ x, 10 से कम एक सम धनात्मक पूर्णांक है’।
- सभी सम अभाज्य संख्याओं के समुच्चय को समुच्चय निर्माण रूप में निम्न प्रकार से लिखेंगे:
S={ x: x एक सम अभाज्य संख्या है}।
इस समुच्चय को निम्न प्रकार से पढ़ते है- ‘सभी x का समुच्चय जहाँ x एक सम अभाज्य संख्या है’।
(Source – Various books from the college library)
Copyrighted Material © 2019 - 2024 Prinsli.com - All rights reserved
All content on this website is copyrighted. It is prohibited to copy, publish or distribute the content and images of this website through any website, book, newspaper, software, videos, YouTube Channel or any other medium without written permission. You are not authorized to alter, obscure or remove any proprietary information, copyright or logo from this Website in any way. If any of these rules are violated, it will be strongly protested and legal action will be taken.




super